INVERSE FUNCTIONS

All functions have the property where each x has one y-partner.
(Graphically: It passes the "VERTICAL LINE TEST".)
If a certain function also has each y with only one x-partner
(Thus, passing a "HORIZONTAL LINE TEST"), it has an INVERSE!

Prefixes like INV or ARC are sometimes used to denote inverse.
Another form looks like f to the -1 power(Not to be confused with RECIPROCAL).



You might say that the INVERSE
undoes what the orginal function did!

To find the inverse of a function f(x)
switch x and y and THEN solve for y.


f(x) = x cubed
switch
x = y cubed
solving for y
y = cube root of x
y = x raised to 1/3 power

(We had to invent the symbols for cube root and 1/3 power.)
The graph of the inverse of f(x) we can graph f(x) and then
rotate this graph through y = x (thus, switching x and y)

Inverse Trig:

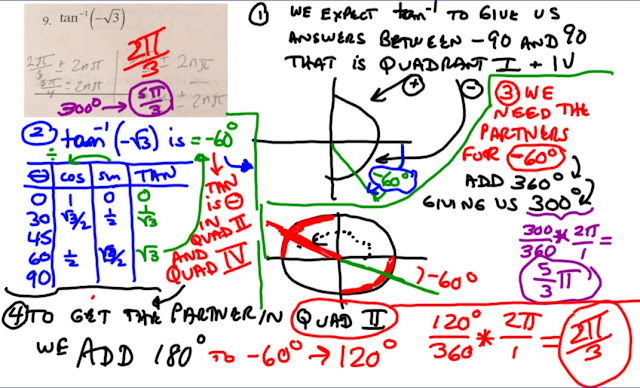INVERSE TRIG:

How to find the partners

for 22 degrees.