SLOPE (of a LINE)

FINDING the EQUATION of a LINE

What is this SLOPE THING?

Suppose we want to buy some T-shirts.

We are told that each T-shirt costs the

same amount. There is a charge

(an initial fee)

for setting up the logo for the order.

The price for 10 shirts would be $93
and 100 shirts would cost $633.

What is the COST for each shirt?

Dollars per Shirt!

(The SLOPE OF A LINE)

The difference in cost 633 minus 93 is $540.

$540 divided by 90 shirts gives

a RATE (Cost per Shirt) of $6.00 per shirt.

Why then do 10 shirts at $6 each cost $93?

Because there is a set-up fee of $33.

The Cost formula that Mr. Smith uses is:

Total Cost = ( $6 ) times # of shirts PLUS $33

We could represent this with the equation:

y = 6 x + 33

(Note: y depends on x for its value!)

$$$$$$$$$$$$$$$$$$$$$$$$$$$$$$$

Take a look at the set of ordered pairs:

{ (3,11) (4,13) (5,15) (6,17)}

We notice that when X changes one unit

(as it does from 3 to 4), Y changes 2 units

(11 to 13). As X changes 2 units (3 to 5),

Y changes 4 units (11 to 15).

NOTE:

We are not saying that the values for y

are twice as big as the values for x.

We are talking about changes in y and x.

Y's change is 2 units for each 1 unit of change in x.
We call this the RATE OF CHANGE OF Y

WITH RESPECT TO X. Graphing these points

onto the xy-plane and connecting the points

with a line we have what is called a line

with SLOPE of 2. The EQUATION that these

points SATISFY is y = 2x + 5. Notice the

SLOPE of 2 is situated next to x.

Picking a point on the graph and moving

3 units to the RIGHT (RUN)

and then moving

6 units UP (RISE) we will land

us back onto the line.

The RISE is 6 and the RUN is 3.

the FRACTION (RATIO)

RISE/RUN EQUALS 2.

One definition for the slope of a LINE

is the RATIO

m = RISE/RUN.

How about the graph of the equation

y = (3/4)x - 1

Some of the ordered pairs that satisfy

this equation are {(4,2) (8,5) (12,8) ...}

When x changes 4 units, y changes 3 units.

The RATIO of 3 to 4 represents the

change in y with respect to the change in x.

THE CHANGE IN Y over the CHANGE in x is 3/4.

The slope of the graph of this equation is 3/4.

The SLOPE of the graph of the solutions to:

(i) a LINEAR FUNCTION f(x) = mx + b

(ii) an equation in the form Ax + By = C

(iii) or in the form y = mx + b

..........SLOPE is the rate that y changes

with respect to a change in x

..........In other words,

m = (y2-y1) / (x2-x1)

or m = change of y divided by the change in x

-----the values for x change by (x2-x1)

and the corresponding y's change by (y2-y1)

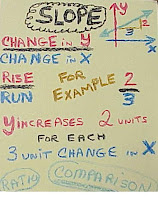
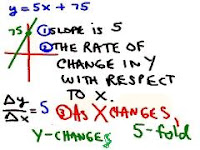
LINK: Back to Contents